How do you solve 6sinx+3cotx-3cscx=0 if 0<=x<2pi?

Question

1679 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-05-03T06:01:07

The only solutions on the given interval are #x=0,(2pi)/3,(4pi)/3#.

To solve the equation, we'll need these identities:

#cotx=cosx/sinx#

#cscx=1/sinx#

#sin^2x+cos^2x=1#

#sin^2x=1-cos^2x#

Now, here's the actual problem. The end strategy is to solve it like a quadratic:

#6sinx+3cotx-3cscx=0#

#2sinx+cotx-cscx=0#

#2sinx+cosx/sinx-1/sinx=0#

#2sin^2x+cosx-1=0#

#2(1-cos^2x)+cosx-1=0#

#2-2cos^2x+cosx-1=0#

#-2cos^2x+cosx+1=0#

#2cos^2x-cosx-1=0#

#(2cosx+1)(cosx-1)=0#

#cosx=-1/2,cosx=1#

Taking a look at the unit circle, we see that #cosx=1# only at #0# radians, and #cosx=-1/2# at #(2pi)/3# and #(4pi)/3# radians:

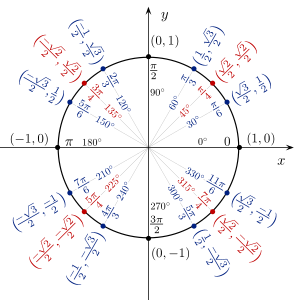
enter image source here

So the solutions on the given interval are:

#x=0,(2pi)/3,(4pi)/3#

Hope this helped!