How do you find the area of a triangle?

The formula for the area of a triangle is #h*w-:2#, where #h# represents #"height"# and #w# represents #"width"# (this can also be referred to as the "base" or "base length").

For example, here we have a right triangle that has a height of #4# and a width of #6#:

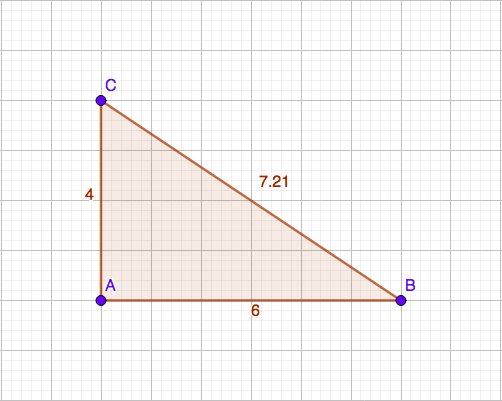
Imagine another triangle, identical to this one, put together with triangle ABC to form a rectangle:

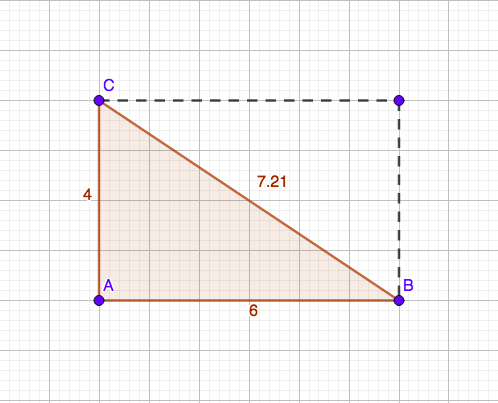
Here we have a rectangle with a height of #4# and a base width of #6#, just like the triangle. Now we find the area of a rectangle by using the formula #h*w#:

#4*6=24#

Now we know the area of the rectangle is #24"cm"^2#, assuming that each square is a cubic centimetre.

So if the area of the rectangle is #24"cm"^2# and the area of triangle ABC is half of that of the rectangle (as shown in the second image), then the area of the triangle is half of that of the rectangle; #12"cm"^2#.

So to find the area of a triangle, the formula is #l*w-:2#.

This expression works with other types of triangles too, not just right angle triangles. For example:

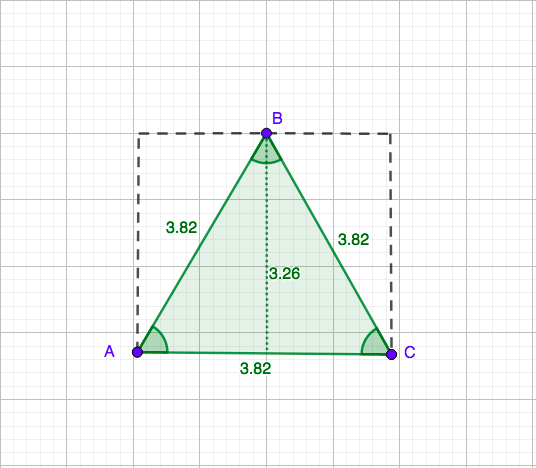
A trick that I use to remember the formula is to draw a square/rectangle around the triangle and use that to find the area.

Hope this helped :)