How do you find the volume of a solid of revolution using the disk method for #y= 3/(x+1)#, #y=0#, #x=0#, #x=8# revolved about the #x#-axis?

Question

The question I am having trouble with is:

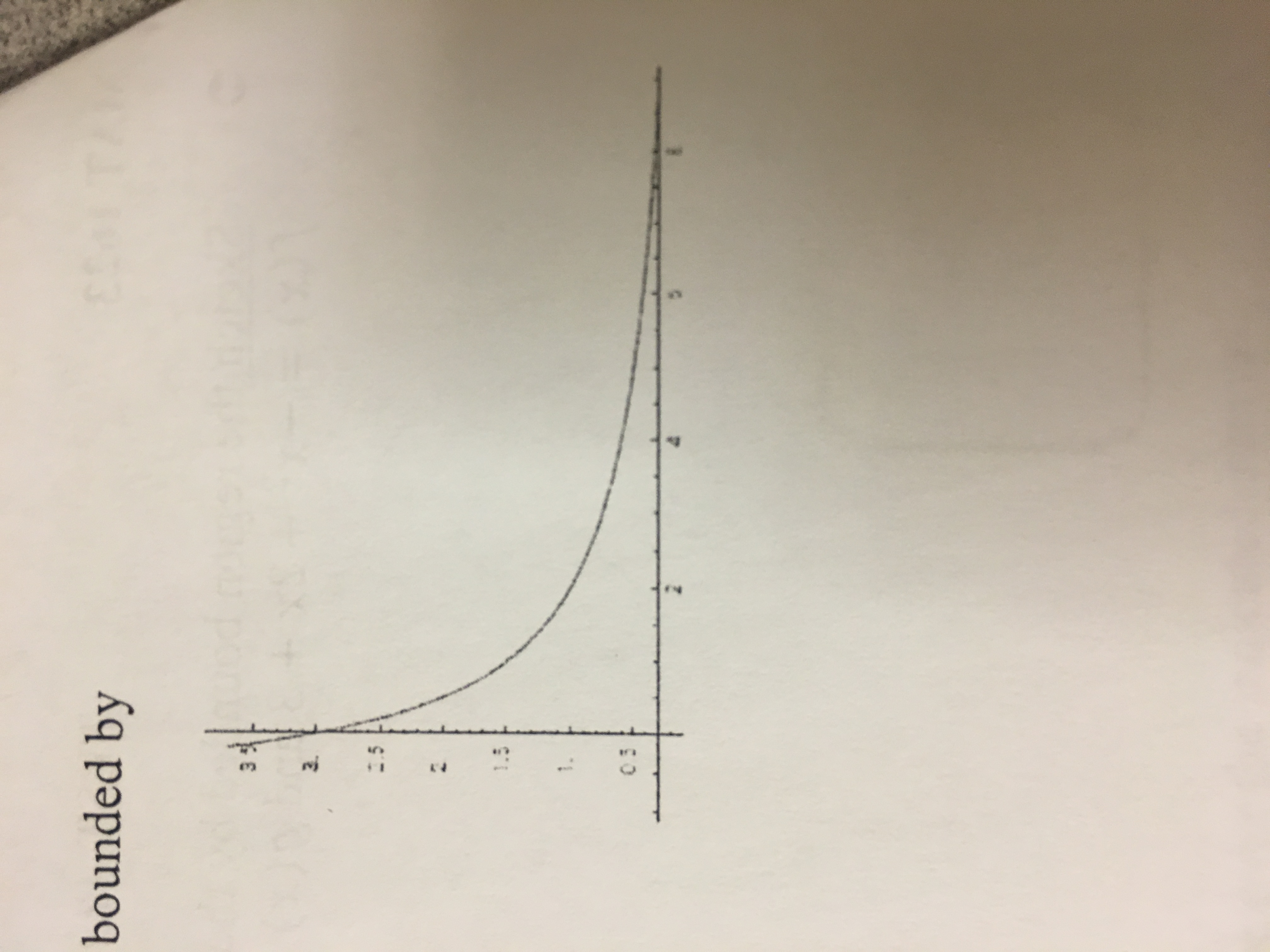
Find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the x-axis . (Using the disc method.)
#y= 3/(x+1)#, #y=0#, #x=0#, #x=8#

2303 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-04-08T15:50:39

Please see below.

Here is a graph of the region:

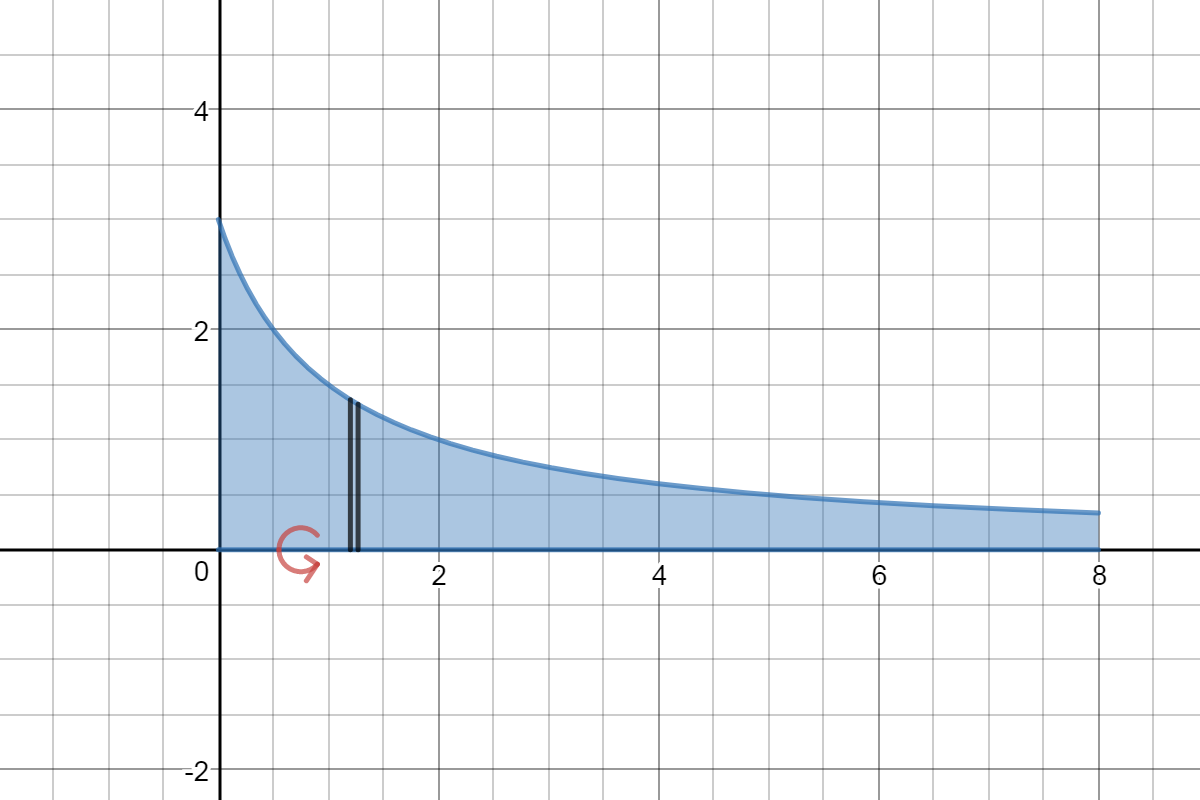
enter image source here

In order to use disks, a representative slice has been taken perpendicular to the axis of revolution. (In this case the axis is a horizontal line.)

For a disk we get representative volume

#pi r^2 " thickness"#

We have taken the slice at some value of #x# and
the thickness is #dx#

In this case, #r = # the #y#-value on the curve, so
#r = 3/(x+1)#

The representative slice has volume: #pi(3/(x+1))^2dx#

The values of #x# vary from #0# to #8#, so the volume of the solid is:

#V = int_0^8 pi(3/(x+1))^2dx = 9pi int_0^8 1/(x+1)^2 dx =8pi#