Question #fd431

If you don't immediately know what to do, ALWAYS draw a diagram.

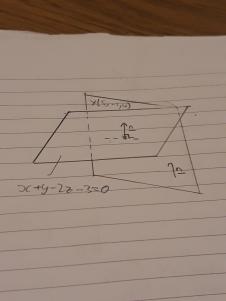
Here, I have the plan given, and the one intercepting it. I've marked a normal vector to the plane given, #uln#, as well as the point P (which I marked as X so sorry about that).

If the two planes are perpendicular, then the normal vector to one plane will be a vector parallel to the other plane. Also, the normal vector to each plane will be perpendicular to each other, and dot product to zero.

Let #uln# be the normal vector to the given plane.

#uln=((1),(1),(-2))#

To find a vector perpendicular to this one:

Let #ulm# be a vector normal to the desired plane.

Let #ulm=((m_1),(m_2),(m_3))#

#uln*ulm=0#

#m_1+m_2-2m_3=0#

There are no unique solutions to this, so let's find a perpendicular vector.

Let #m_3=1#

#m_1+m_2=2#

Let #m_2=-1 => m_1=3#

#:. ulm=((3),(-1),(1))#

To check this is normal to the desired plane,

#ulm*uln=((3),(-1),(1))*((1),(1),(-2))#
#=3-1-2#
#=0#

Since we now have a vector normal to our desired product, we can use the scalar product equation of a plane:

#ulr*ulm=ulp*ulm#
#ulr*((3),(-1),(1))=((5),(-1),(4))*((3),(-1),(1))#

#ulr*((3),(-1),(1))=15+1+4#

#ulr*((3),(-1),(1))=20#

In Cartesian form:

#3x-y+z-20=0#