Vectors Please Help ( What is the direction of vector A + vector B?)

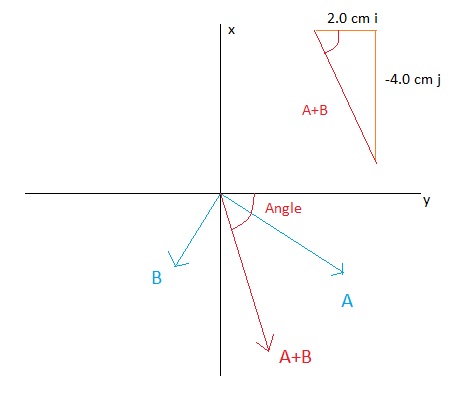
Not drawn to scale

Sorry for the crudely drawn diagram but I hope it helps us see the situation better.

As you have worked out earlier in the question the vector:

#A+B=2i-4j#

in centimeters. To get the direction from the x-axis we need the angle. If we draw the vector and split it up into its components, i.e. #2.0i# and #-4.0j# you see we get a right angled triangle so the angle can be worked out using simple trigonometry. We have the opposite and the adjacent sides. From trigonometry:

#tantheta = (Opp) /( Adj) implies theta=tan^-1((Opp) /(Adj))#

In our case the side opposite the angle is #4.0cm# so #4.0cm# and the adjacent side is: #2.0cm# so:

#theta = tan^-1(4.0/2.0)=63.425^o#

Obviously this is anti-clockwise so we must put a minus in front of the angle #-> -63.425#

If the question is asking for the positive angle going clockwise around the diagram then simple subtract this from #360^o#

#-> 360-63.425=296.565^o#

It looks like your answer for e is wrong and perhaps you have not found an answer for f. So I will help with both.

Note: I am using the angle measuring method in which you start at the +x axis and circulate counterclockwise to the vector. So the +y axis is at #90^@# and the minus y axis is at #270^@#. Ref:
http://chortle.ccsu.edu/VectorLessons/vch05/vch05_3.html

e. From your work, #vec(A) + vec(B) = 2 " cm " hati - 4 " cm " hatj#. That puts the vector in the 4th quadrant. Draw the vector with the arrowhead at x=2, y=-4.

Let's calculate the angle #theta_e# between the -y axis and the vector. The length of the side opposite is 2 cm and the side adjacent is 4 cm.

#tan^-1(2/4) = 26.5^@#

The -y axis is already #270^@# counterclockwise from the +x axis, so the answer to e is #270^@+26.5^@ = 296.5^@#.

f. From your work, #vec(A) - vec(B) = 4 " cm " hati + 0 " cm " hatj#. Therefore the resultant lies along the x axis. That is an angle of #0^@#.

I hope this helps,
Steve