Question #5c760

Question

818 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-01-25T21:59:42

(a)

#sf(v_0=8.9color(white)(x)"m/s")#

(b)

She will just make the bank at 10 m.

(a)

The expression for range is given by:

#sf(d=(v_0^2sin2theta)/g)#

#:.##sf(v_0^2=(dg)/(sin2theta)=(8.0xx9.81)/(sin90)=78.48)#

#sf(v_0=sqrt(78.48)=8.86color(white)(x)"m/s")#

(b)

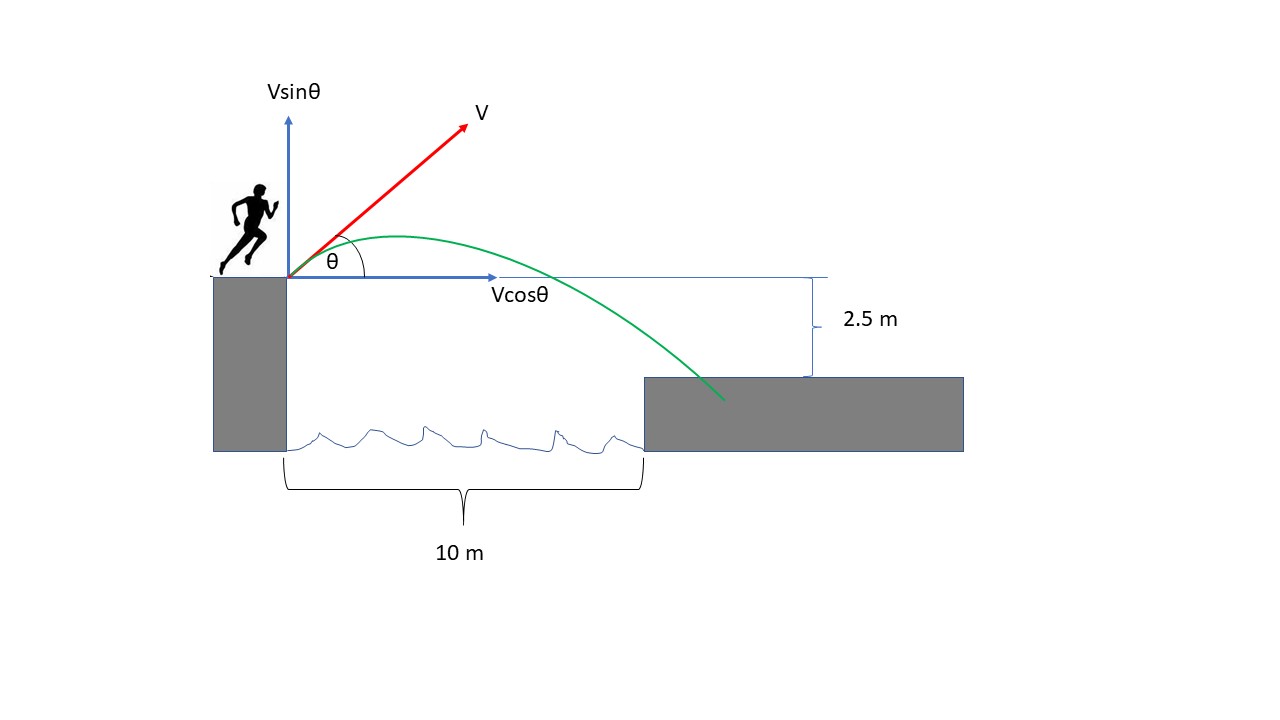
MFDocs

The key to this is to find the time of flight, which is common to both the vertical and horizontal components of motion:

Using the equation of motion:

#sf(s=ut+1/2at^2)#

If we set the launch point as the origin this becomes:

#sf(-2.5=vsinthetat-1/2"g"t^2)#

#sf(-2.5=8.86sin45t-1/2xx9.81t^2)#

#sf(-2.5=8.86xx0.707t-4.905t^2)#

#sf(4.905t^2-6.26t-2.5=0)#

We can use the quadratic formula to solve this. Ignoring the -ve root this gives:

#sf(t=1.596color(white)(x)s)#

The horizontal component of velocity is constant and is equal to #sf(v_0cos45)# so we can write:

#sf(d=vxxt=8.86cos45xx1.596=10color(white)(x)m)#

She just makes it.