For a spontaneous process in an isolated system, the change in entropy is positive. Why? Why not?
2 Answers
It's true
Explanation:
Because the second principe of thermodynamic say that in a isolated system the change in entropy can only be positive or equal zero
if it's equal to zero so the process is reversible, but to be reversible the process need to be in equilibrium for every small (infinity small in theory) change in the process
In your case there is no equilibrium between the start state and the final state because your process is spontaneous
Yes, that is true, but only guaranteed for the system, not the surroundings. It is also important to note that since the system is isolated,
[If the process was instead reversible (and consequently not spontaneous), then it's possible that
A spontaneous process can only be reversed if a different path is taken than what was used going forward (since the microscopic reverse is nonspontaneous). Hence, this process must be irreversible.
Take this spontaneous reaction for example:
 http://www.chem.ucla.edu/
http://www.chem.ucla.edu/
The reverse must be nonspontaneous, since the role of products and reactants are switched.
An irreversible process (any real process) is inefficient. (A truly reversible process keeps the entropy of the universe constant.)
 https://chem.libretexts.org/
https://chem.libretexts.org/
Thus, some energy disperses as a result that was not used for the process itself.
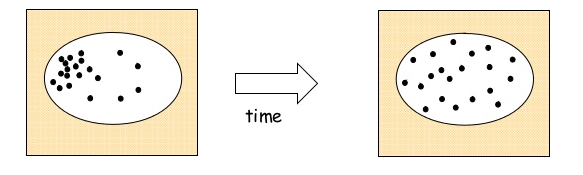 https://www.slideshare.net/erletshaqe1/lecture-19-entropy
https://www.slideshare.net/erletshaqe1/lecture-19-entropy
Since the system is isolated, no heat can escape it (the process is thus adiabatic), so when this flow of energy disperses inside the system, the entropy of the system increases, i.e.
Hence, the entropy of the system must increase for a spontaneous process in this isolated system.
And this occurs until the system has reached equilibrium, which is when the system entropy has maxed out, subject to the constraints placed upon the system.
In other words, the distribution of states is as spread out as it can get, and there is no macroscopic indication of an ongoing process.

