How find the values of x when given y?

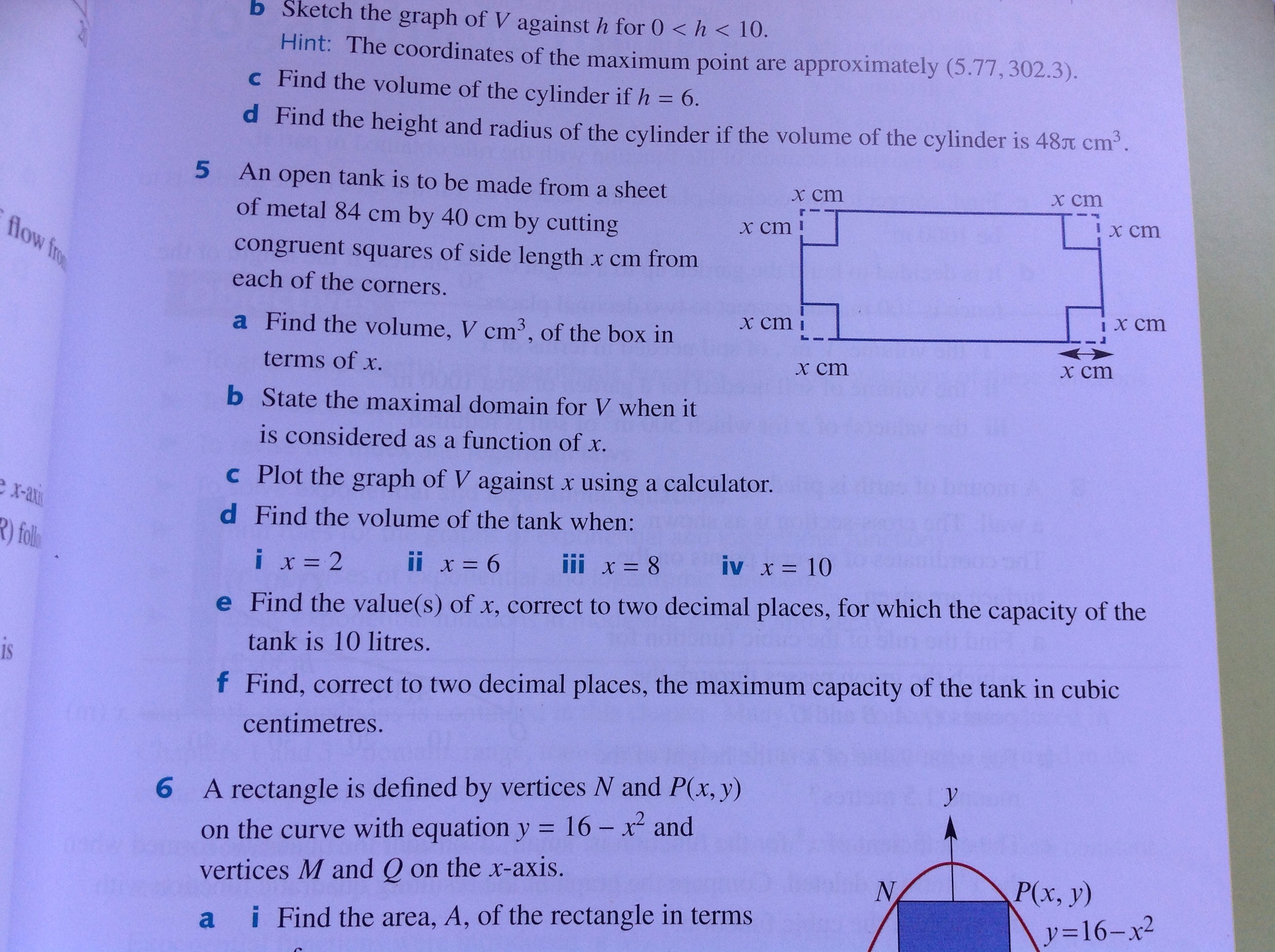
enter image source here
Can someone please explain to me how to do question 5e? Thank you!

1 Answer
Leland Adriano Alejandro
Dec 17, 2017

a. V=length * width * height#=(84-2x)*(40-2x)*x#
b. Maximum value of #x=8.537729734#
f. Maximum volume #V=13,098.71 cm^3#

Explanation:

The solution is as follows

if square of size x is to be made at the corners then the dimension of the box is
length #=(84-2x)#
width #=(40-2x)#
height #=x#
volume #=(84-2x)*(40-2x)*x#

(a) volume #V=4x^3-248x^2+3360x#

by the Calculus tool using derivatives

#V'=12x^2-496x+3360x#
set #V'=0#

Solve the quadratic equation
#12x^2-496x+3360x=0#

and #x_1=32.7956036# and
#x_2=8.537729734#

b. Maximum value of #x=8.537729734#

which gives the maximum volume

(c) For the graph
https://www.desmos.com/calculator/rzv6yekowm

(d) #x=2# and #V=4(2)^3 -248(2)^2+3360(2)=5760#
#x=6# and #V=4(6)^3 -248(6)^2+3360(6)=12096#
#x=8# and #V=4(8)^3 -248(8)^2+3360(8)=13056#
#x=10# and #V=4(10)^3 -248(10)^2+3360(10)=12800#

(e) Solve the equation with #V=10000#
#4x^3-248x^2+3360x=10000#
#4x^3-248x^2+3360x-10000=0#

Using scientific graphics calculator the values are

#x_1=44.319#
#x_2=13.503#
#x_3=4.177#

(f) Maximum volume
#V_max=4(8.53772973)^3-248(8.53772973)^2+3360(8.53772973)#

#V_max=13,098.71#

God bless..... I hope the explanation is useful.

Related questions
Impact of this question
3645 views around the world
You can reuse this answer
Creative Commons License