If #sin theta = 21/29#, what is #cos theta# and #tan theta#?

1 Answer
Dwight
Nov 14, 2017

#costheta= 20/29#

and #tantheta=20/21#

details follow...

Explanation:

It is common to express the three trig functions as ratios involving the sides of a right-angle triangle, whose sides are called the adjacent side, the opposite side and the hypotenuse.

In the diagram below, these are a, b, and c respectively.

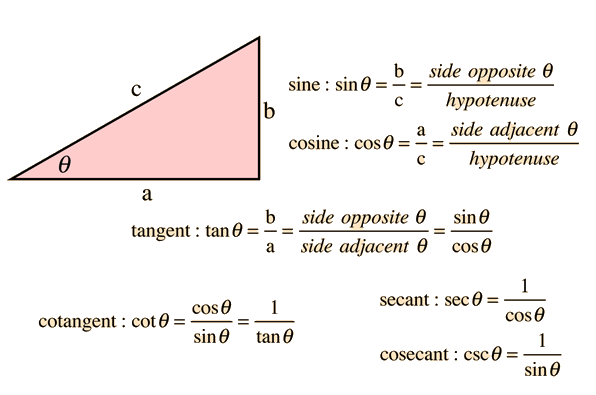
hyperphysics.phy-astr.gsu.edu/hbase/imgmth/ttrig.gif

As the diagram shows, each trig function can be expressed as a ratio:

#sintheta=b/c#, so in your problem, we can assign the value 21 to #a#, and 29 to #c#.

By the Pythagorean theorem,

#b=sqrt(c^2-a^2) = sqrt(29^2-21^2) = sqrt400 = 20#

So, #costheta= a/c = 20/29#

and #tantheta=b/a = 20/21#

Related questions
Impact of this question
23294 views around the world
You can reuse this answer
Creative Commons License