Help me in solving this question?

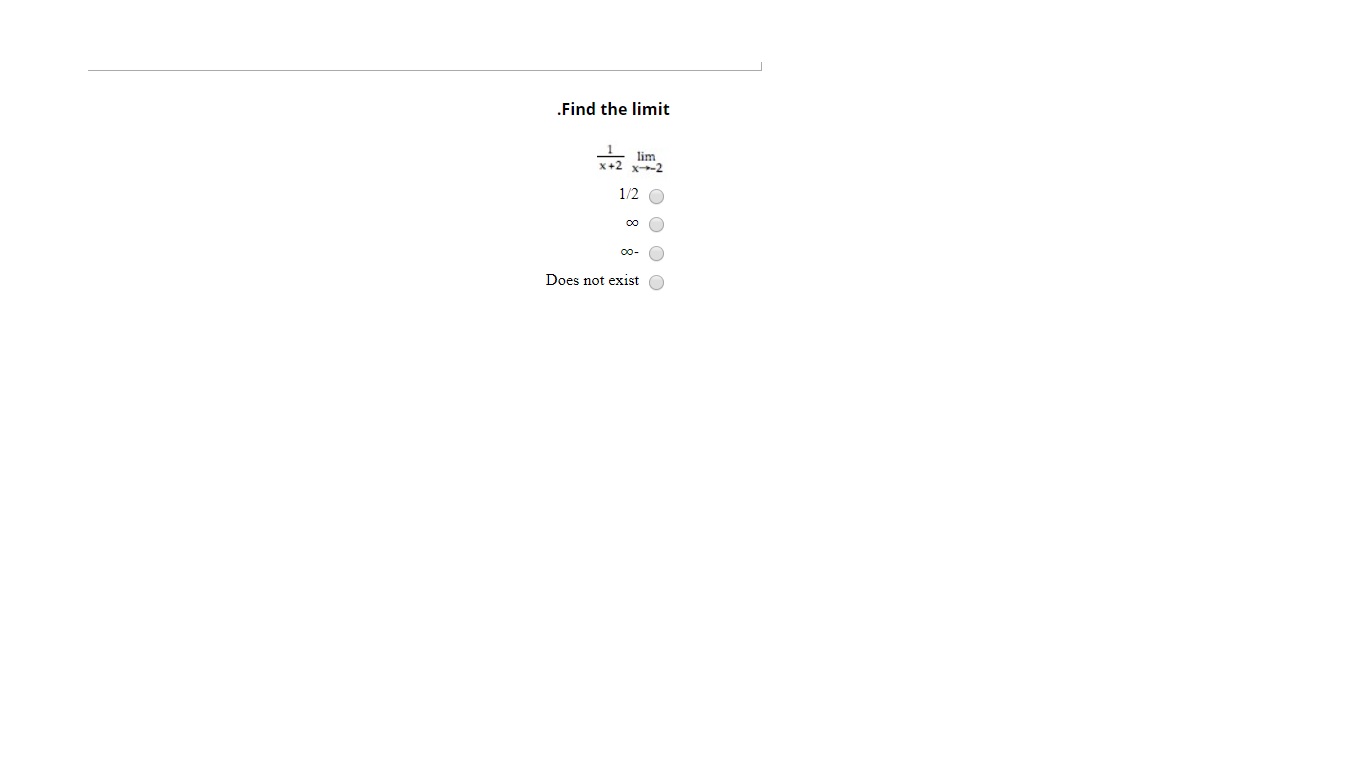
enter image source here

1 Answer
Psykolord1989 .
Oct 12, 2017

The limit does not exist, see explanation below.

Explanation:

We know the function does not exist at the desired point, but it is possible that a LIMIT does.

In order to calculate the limit here, it behooves us to consider what would happen if we were very slightly to the left or the right of the desired x-value. Thus, we consider an arbitrarily small positive number, defined as #epsilon#, and look at what we would receive if #x=-2+-epsilon#.

If #x=-2+epsilon...#

#1/(x+2) = 1/(-2+epsilon+2) = 1/epsilon#

Thus, as epsilon goes to zero, the function will approach positive infinity on the right (i.e. the right hand limit is #oo#).

On the other hand, if #x=-2-epsilon#

#1/(x+2) = 1/(-2-epsilon+2) = 1/-epsilon = -1/epsilon #

Thus, as #epsilon->0#, the function approaches #-oo# from the left. This means that our left and right hand limits are not equal, and thus the limit for the overall function does not exist at the given point.

Related questions
Impact of this question
873 views around the world
You can reuse this answer
Creative Commons License