Find all of the points on the curve #y = x^3 +5x^2# where the tangent line is parallel to the line #y = 11x−π#.?
2 Answers
The line given
Set the derivative of the given curve equal to
Complete the square to solve the quadratic:
A number squared cannot equal a negative number, so there are no points where the tangent line has a slope of
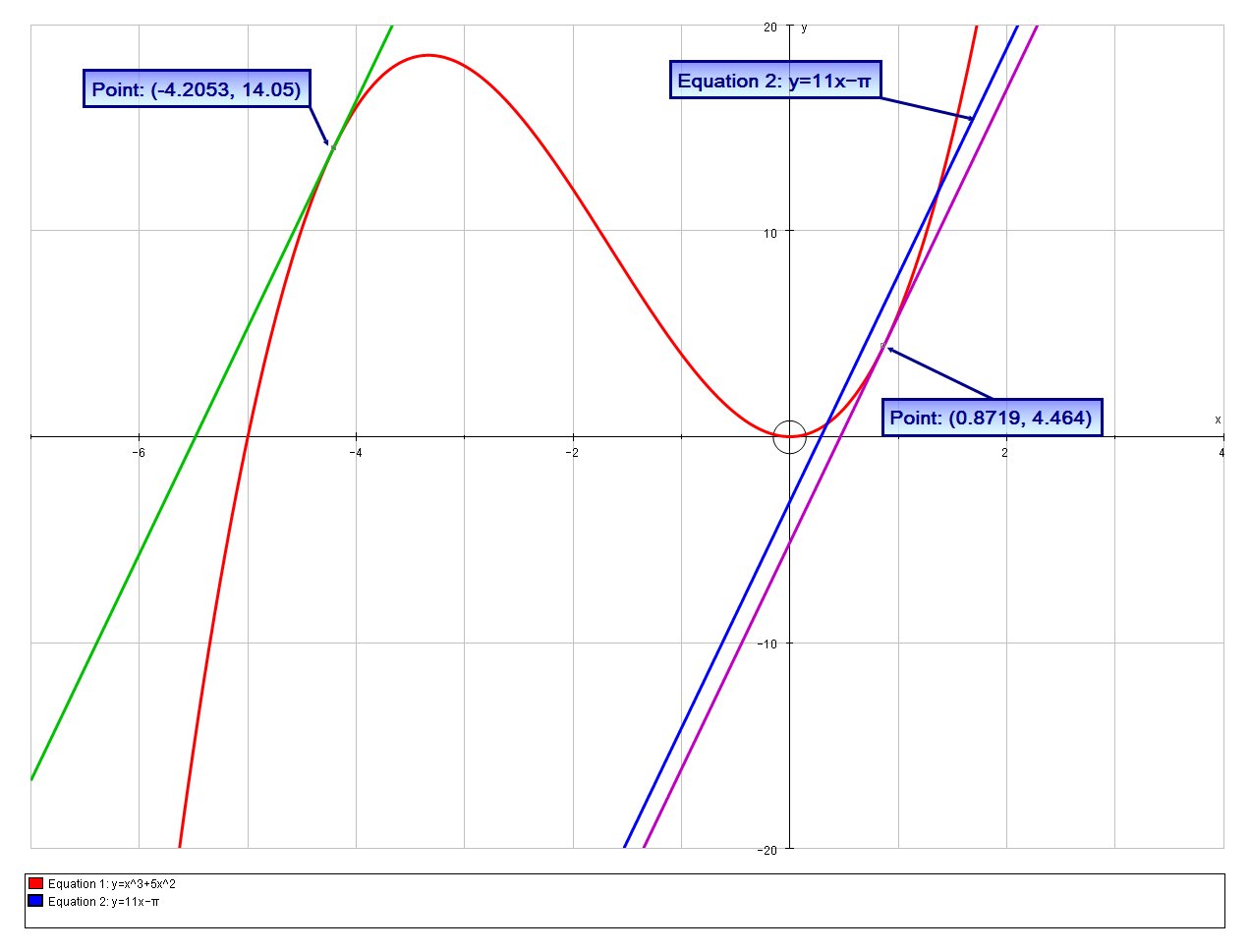
# (-4.2053, 14.0544) # and#(0.8719,4.6441) # (4dp)
Explanation:
The gradient of the tangent to a curve at any particular point is given by the derivative of the curve at that point. (If needed, then the normal is perpendicular to the tangent so the product of their gradients is
We have:
# y=x^3+5x^2 #
Then differentiating wrt
# dy/dx = 3x^2+10x#
Now comparing the the line equation
So any tangent to our curve that is parallel must have this same gradient. This will occur if and only if:
# dy/dx = 11 #
# :. 3x^2+10x = 11#
# :. 3x^2+10x - 11 = 0#
We can solve this quadratic equation using the quadratic formula, giving:
# x = (-10 +- sqrt(10^2-4*3*(-11)))/(2*3) #
# \ \ = (-10 +- sqrt(100+132))/(6) #
# \ \ = (-10 +- sqrt(232))/(6) #
# \ \ = (-10 +- 2sqrt(58))/(6) #
# \ \ = -5/3+- 1/3sqrt(58) #
# \ \ = -4.2053, 0.8719# (4dp)
Using the curve equation we can find the full coordinates:
# x = -4.2053 => y = 14.0544 #
# x= \ \ \ \ \ 0.8719 => y = 4.6441 #
So there are two coordinates on the curve:
# (-4.2053, 14.0544) # and#(0.8719,4.6441) # (4dp)
We can confirm this is correct graphically: