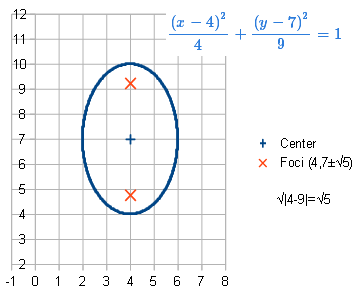
What does #(x-4)^2/4+(y-7)^2/9=1# represent?
1 Answer
Jan 21, 2017
Looks like a vertical ellipse, center (4,7), foci (4, 7±√5).
Explanation:
The equation is already in the standard form showing the center (4,7), semi-major and semi-minor values (3 & 2). The main parameter derived from this is the distance between the center and either focus, which is given by the square root of the absolute value of the difference between the semi-major and a semi-minor axes. Because 3>2, the ellipse is oriented vertically, that is, the foci have the same x-coordinate. The sum of the distance from any point on the ellipse to the two foci is 6.