How do you use a power series to approximate # int_0^1xtan^-1xdx #?

Let # I = int_0^1xtan^-1xdx #

The power series for #tan^-1x# is as follows:

# tan^-1x = x-x^3/3+x^5/5-x^7/7 +x^9/9-x^11/11+x^13/13 - ... #

And so we get the integrand by multiplying by #x# to give:

# xtan^-1x = x^2-x^4/3+x^6/5-x^8/7 +x^10/9-x^12/11+x^14/13 - ... #

And so replacing the integrand with the power series we have:

# I ~~int_0^1 x^2-x^4/3+x^6/5-x^8/7 +x^10/9 - ... dx #

Integrating each term gives us:
# I =[ x^3/3-x^5/(3.5)+x^7/(5.7)-x^9/(7.9) +x^11/(9.11) - x^13/(11.13)...]_0^1 #

Applying the limits gives us:
# I =1/(1.3)-1/(3.5)+1/(5.7)-1/(7.9) +1/(9.11) - 1/(11.13) + ... #

We will need to take sufficient terms to get an answer that is stable to 4dp, which I will perform in Excel as follows;

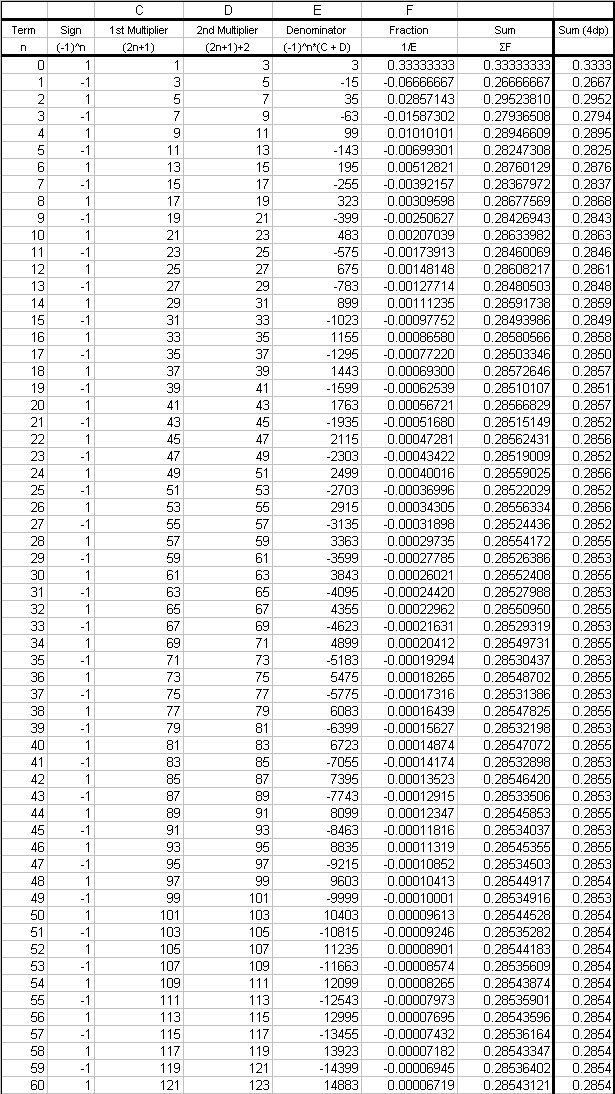
Due to the oscillating natures of the power series it takes a significant number of terms to get a stable answer, but we can conclude that
# I ~~ 0.2854 # (4dp)

#int_0^1x(tan)^-1xdx#
let#(tan)^-1x=theta#
or #tantheta=x# hence# dx=sec^2theta d theta#
then the integral reduces to #int_0^(pi/4) theta tantheta sec^2 theta d theta#
now we use the integration by parts
so the integral=#theta int_0^(pi/4)tan theta sec^2 theta d theta-int_0^(pi/4)(1 inttan theta sec^2 theta d theta)d theta#
=#int_0^(pi/4)tantheta d( tantheta)# -#int_0^(pi/4)((inttantheta d( tantheta)))d theta#
now the indefinite integral #inttantheta d( tantheta)#=#1/2 tan^2 theta#
so for the limit we get the value of first integral=#pi/8#

similarly the indefinite integral # int tan^2 theta d theta#=#int(sec^2 theta -1) d theta#=#tan theta-theta#
so for the req. limit we ge#t =1/2-pi/8#
so the ans. is =#pi/8-(1/2-pi/8)#
=#pi/4-1/2#