Question #bdd78
1 Answer
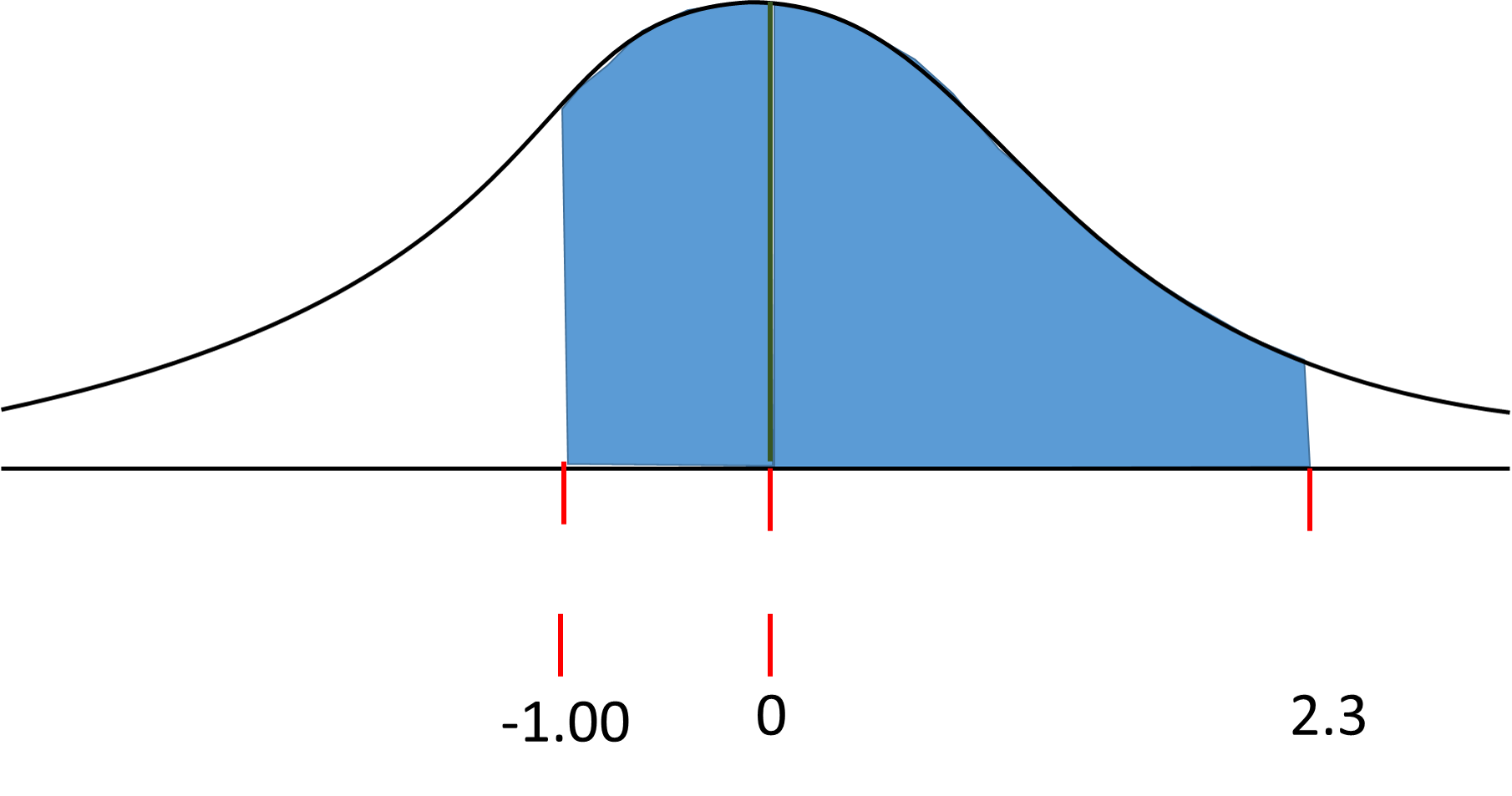
Area[between z -1 and z = 2.3] = 0.3413 + 0.4893 = 0.8306
Explanation:
Area under the standard normal curve represents a total probability of one. 0.5 probability is represented by one half of the curve.
By converting the x - values of a Normal Distribution, corresponding z - values are obtained. When the curve is referenced by z-values, it is called a Normal Probability Distribution or Standard Normal Distribution. Then for any given range of z-values, we can find the probability value using the Probability table. The table is given in all the statistics books.
In the table you find z-values along the left extreme column[First column]. It is to be read in combination with the top most column [First Row]. What is given inside these two are the Probability values governed by the area between z = 0 and z = any given value.
You will understand this when we solve your problem.
Look at the diagram. The coloured area represents the probability value.
The area we have to find is [between z = -1 and z = 2.3]
This is equal to area [between z = 0 and z = -1] + area [between z = 0 and z = 2.3]
You have to break the whole area like this because the table gives probability value for the positive values of z. It represents one half of the curve. What is applicable to the right hand side is equally applicable to the left hand side also.
Now we shall find the area [between z = 0 and z = -1]. It is 0.3413
Then we shall find the area [between z = 0 and z = 2.3]. It is 0.4893
This is the steps involved in solving the problem
Area[between z = -1 and z = 2.3] = area [between z = 0 and z = -1] + area [between z = 0 and z = 2.3]
Area[between z -1 and z = 2.3] = 0.3413 + 0.4893 = 0.8306