Iodine-131 is a radioactive isotope with a half-life of 8 days. How many grams of a 64 g sample of iodine-131 will remain at the end of 24 days?

The nuclear half-life of a radioactive isotope tells you how much time must pass in order for half of the atoms present in an initial sample to undergo radioactive decay.

In essence, the half-life tells you at what time intervals you can expect an initial sample of a radioactive isotope to be halved.

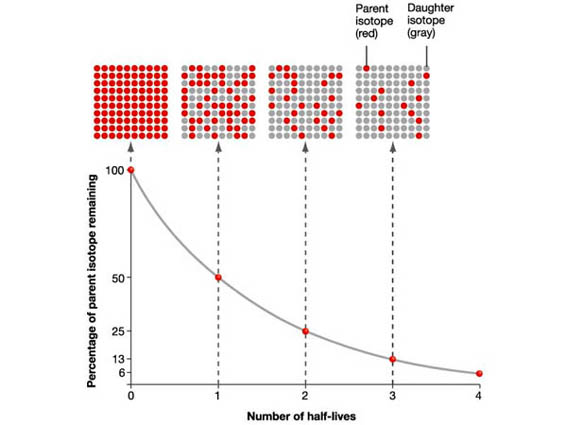
In your case, iodine-131 is said to have a half-life of #8# days. This means that with every #8# days that pass, your sample of iodine-131 will be halved.

If you take #A_0# to be the initial sample of iodine-131, you will be left with

#1/2 * A_0 = A_0/2 -> # after the passing of one half-life

#1/2 * A_0/2 = A_0/4 -># after the passing of two half-lives

#1/2 * A_0/4 = A_0/8 -># after the passing of three half-lives
#vdots#

and so on.

You can thus say that the amount of iodine-131 that remains undecayed, #"A"#, after a period of time #t#, will be equal to

#color(blue)(|bar(ul(color(white)(a/a)A = A_0 * 1/2^ncolor(white)(a/a)|)))#

Here #n# represents the number of half-lives that pass in #t#.

In your case, you have

#n = (24 color(red)(cancel(color(black)("days"))))/(8color(red)(cancel(color(black)("days")))) = 3#

This will get you

#A_"24 days" = "64 g" * 1/2^3#

#A_"24 days" = color(green)(|bar(ul(color(white)(a/a)"8 g"color(white)(a/a)|)))#

Half Life follows first Order kinetics

This graph shows what is going on during the decay

As you can see from the graph the half life is 8 days

Lets do the math and verify it with the graph

Recall

# t_(1/2) = ln2/k#

#8 = ln2/k#

#k = ln2/8#

With this you can solve most problems regarding the above data

#ln(A_o/A_t) = kt#

#ln(A_o/A_t) = ln2/8 * 24 = 3 ln2 = ln 2^3 = ln8#

#(A_o/A_t) = 8#

Now

Greater the concentration of a sample greater the mass in in it

#C prop M#

So

#(A_o/A_t) = (m_o/m_t) = 8#

#"we have "m_o = 64 g"#

#64/m_t = 8#

#=> m_t = 8g#

Verifying with the graph

#m_("24 hours") = 12.5 % m_o = 12.5 *10^-2 *m_o#
# =12.5 *10^-2 *64 = 8g#

Hence verified