Find the area of the shaded region (green) knowing the side of square is #s = 25 cm#?

Use the formula for area of a triangle: #a = (b xx h)/2#

#a = (25 xx 25)/2#

#a = 312.5#

Therefore, the area of the shaded half square is 312.5 square centimetres.

I could be wrong about it being impossible to find the area of the complete shaded area. I have flagged my answer so that other contributors can think about it.

Hopefully this helps.

The area of the green space can be calculated as the difference between the area of the square and the area of the semicircle. The area of the square is easy to calculate as we are given the side length, and we know the area of the semicircle is half the area of the circle with the same radius. Then, to solve the problem, the main task is to find #r#.

The picture seems to indicate that the diagonal of the square is tangent to the semicircle. We will operate based on that assumption.

We can label the picture as follows:

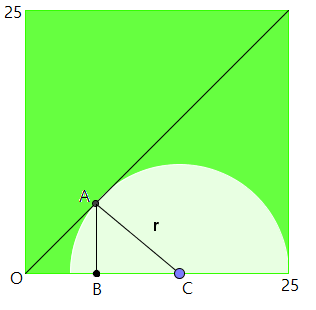
Imposing the image on a coordinate plane with the lower left corner of the square at #(0,0)#, let the coordinate for #A# be #(x_0,y_0)#. Noting that the diagonal of the square is a segment of the line #y=x# we can rewrite #A=(x_0,x_0)#.

Because the diagonal is tangent to the circle and #bar(AC)# is a radius of the circle, their intersection forms a #90^@# angle, and thus we have #angleOAC = 90^@#

Then, by symmetry, #triangleABC# is a #45-45-90# right triangle, meaning #bar(AB) = bar(BC)#. But we know #bar(AB)=x_0# from the coordinates of #A#, and so, applying symmetry again, we have #bar(BC) = bar(OB) = x_0#.

As the sum of those line segments together with a radius of the semicircle gives us the base of the square, we have

#2x_0+r=25#

#=> 2x_0 = 25 - r#

#=> 4x_0^2 = r^2 - 50r + 625#

Next, noting that #bar(AC) = r#, we can use the Pythagorean theorem to obtain #r^2 = x_0^2+x_0^2=2x_0^2#. Substituting this into the above equation gives us

#2r^2 = r^2-50r+625#

#=> r^2+50r-625 = 0#

Applying the quadratic formula, we get

#r = (-50+-sqrt(5000))/2 = -25+-25sqrt(2)#

As we know #r > 0# we can discard the negative result, leaving us with

#r = 25sqrt(2)-25=25(sqrt(2)-1)~~10.355#

Now, given the area of the square as #25^2# and the area of the semicircle as #1/2(pir^2)# we can substitute in our value for #r# to find the green space to be the difference:

#25^2-(pi(25(sqrt(2)-1))^2)/2 = 625-(625pi(sqrt(2)-1)^2)/2~~456.559#