An object with a mass of #7 kg# is on a surface with a kinetic friction coefficient of # 8 #. How much force is necessary to accelerate the object horizontally at # 4 m/s^2#?

1 Answer
Miguel F.
Mar 25, 2016

Let us apply Newton's Laws to solve this problem.

Explanation:

According to Newton's 2nd Law of Motion (#F=ma#), to get an acceleration of 4 #"m/s"^2#, we need a force:

#F = m cdot a = 7 " kg" cdot 4 " m/s"^2 = 28 " N"#

This would be the force necessary to move the body if there were not any other forces (as friction). However, in our case, a stronger force is required to compensate friction.
So, let us take 28 N as net force.

Now, we are going to find friction force. This equals to:

#F_"friction" = mu cdot N = mu cdot m g = 8 cdot 7 " kg" cdot 9,8 " m/s"^2 =#
#= 548,8 " N"#

So net force will be:

#F_"net" = F_"applied" - F_"friction" = 28 " N"#

We can see next picture to understand it better:

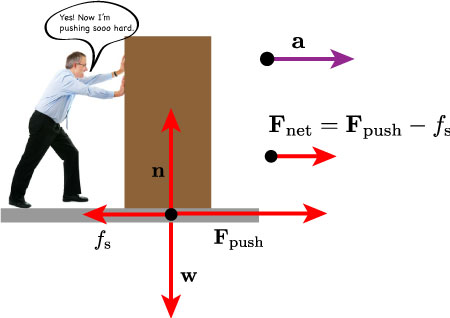
enter image source here

Now, having #F_"net"# and #F_"friction"#, let us find #F_"applied"#:

#F_"applied" = F_"net" + F_"friction" = 28 " N" + 548,8 " N" = 576,8 " N"#

Related questions
See all questions in Frictional Forces
Impact of this question
2115 views around the world
You can reuse this answer
Creative Commons License