What is the arclength of #f(x)=x/e^(3x)# on #x in [1,2]#?

1 Answer
David B.
Feb 19, 2016

Approximately 1.

Explanation:

The arc length is calculated from the following integral:

#L=int_a^bsqrt(1+((df(x))/dx)^2)dx#

which leads us to

#L=int_1^2sqrt(1+[(1-3x)e^(-3x)]^2)dx#

I haven't found an analytic expression for the integral. However, when we plot the function #f(x)# it becomes clear that the function has decayed to very small values.

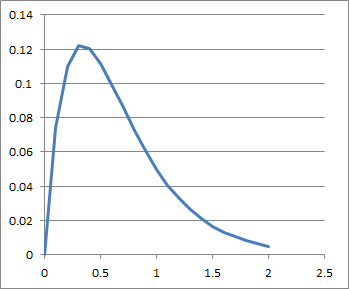
enter image source here

What is deceiving about this graph is that arc-length is calculated in normal Cartesian space, which implies that the scale of #x# and #y# should be equal as in the following version:

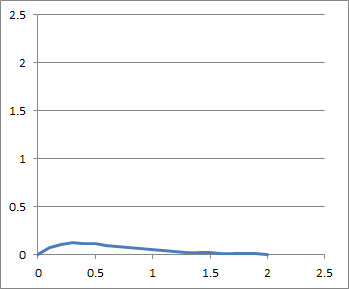
enter image source here

This implies that the actual arc length between 1 and 2 will be very close to the distance on the axis. Doing the integration numerically, one gets

#L~=1.0013...#

which is very close to 1 as expected. A possible refinement would be to approximate the arc length with a straight line between #f(1)# and #f(2)# giving the two points

#(1, 0.050) and (2, 0.005)#

#L~=sqrt(1+(0.050-0.005)^2)=1.0010...#

Related questions
See all questions in Determining the Length of a Curve
Impact of this question
1504 views around the world
You can reuse this answer
Creative Commons License