Question #bf8db

1 Answer
Rui D.
Feb 3, 2016

#63.435^@#

Explanation:

Consider the figure below

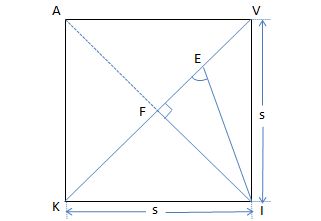
I created this figure using MS Excel

We know that
#3VE=EK# or #EK=3VE#
And
#VE+EK=VK#
Then

#VE+3VE=VK# => #VE=(VK)/4#
Since #VK=sqrt(2)*s# => #VE=sqrt(2)/4*s#

Since #triangle_(AFV)-=triangle_(FIV)-=triangle_(FIK)-=triangle_(AFK)#
#-> FV=(VK)/2# => #FV=sqrt(2)/2*s# (and #FI=FV#)
#-> EF=FV-VE=sqrt(2)/2*s-sqrt(2)/4*s# => #EF=sqrt(2)/4*s#

In #triangle_(EFI)#

#EI^2=EF^2+FI^2=(sqrt(2)/4*s)^2+(sqrt(2)/2*s)^2=(2/16+2/4)s^2=(2+8)/16*s^2# => #EI=sqrt(10)/4*s#

Still in #triangle_(EFI)#, using Law of Sines:

#(EI)/(sin 90^@)=(FI)/(sin F hat E I)# => #sin F hat E I=(FI*1)/(EI)=(sqrt(2)/2*cancel(s))/(sqrt(10)/4*cancel(s))=2*sqrt(2/10)=2/sqrt(5)#
=>#F hat E I=sin^(-1) (2/sqrt(5))=63.435^@#

Related questions
Impact of this question
830 views around the world
You can reuse this answer
Creative Commons License