How do you divide #( 8i+2) / (-i +2)# in trigonometric form?

Tip: this is just a theoretical introductory; you can jump to the text below the image to see the problem solution.

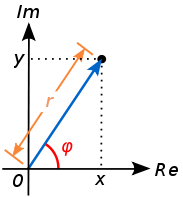
A complex number #z# can be written in many ways:

• Binomial form: #z = x + y cdot "i"#
  where #a# is the real part and #b# the imaginary part.
• Cartesian form: #z = (x, y)#
  just as the binomial form, but written as an ordered pair.
• Polar form: #z = r_phi#
  where #r# is the modulus (or absolute value) of the number and #theta# is the argument.
  -- The modulus is obtained by: #r = sqrt{x^2+y^2}#
  -- The argument is obtained by: #phi= arctan(y/x)#. It must be always between #-pi/2# and #pi/2#
• Cartesian form: #z = r cdot (cos phi+ "i" cdot sin phi)#
• Exponential form: #z = r cdot e^phi#
  where #e# is the exponential.

To sum up, there are two ways to represent a complex number:
- Depending on its coordinates, #x# and #y#.
- Depending on its vectorial magnitudes, #r# and #phi#.

If we want to add and substract complex numbers, we should use cartesian or binomial forms; however, if we want to solve a product or a fraction, we should use polar form, and then transform into the one which interests us.

Let us divide #{8"i"+2}/{-"i"+2}# in trigonometric form.
First of all, we must transform both numbers (numerator and denominator) from binomial to polar form, and then we will transform the result into trigonometric form.

• #8 "i" + 2 = 8.2462_1.33#
• #-"i" + 2 = 2.2361_{-0.46}#

And now, we divide both modulus, and we substract both arguments:

#{8.2462_1.33}/{2.2361_{-0.46}} = 3.6878_1.79#

Finally, we transform it into trigonometric form:
#3.6878_1.79 equiv 3.6878 cdot (cos 1.79 + "i" sin 1.79)#